This post has a lot of pictures and is too long for some email clients; you can read it on the Substack website or in the app.
This is part of a new series about how to help yourself or your kids improve in mathematics. It’s based partly on my own experience of having to learn how to learn mathematics, partly on extensive tutoring experience, and partly on many conversations with homeschooling parents, as well as parents struggling to understand Common Core mathematics. Many future editions are already planned, but feel free to leave suggestions for future editions in the comments (open for paid subscribers) or by email to hollymathnerd at gmail dot com.
I may put future editions behind the paywall; I’m not sure yet. If you want to subscribe to be sure you get them all, this link will give you 10% off. If you can’t afford a paid subscription, email me and I’ll give you a free one.
Part 1: Addition and Subtraction
First, a refresher. Most of you have been using calculators or apps for years now, so I’m writing out a refresher to help. To grasp both addition and subtraction, the first core concept is place value:
Now that we remember how place values work, here is a refresher on addition:
And one on subtraction, including borrowing:
But What ARE Addition and Subtraction?
Addition and subtraction are the mathematical operations for combining or separating. We think of them as opposites, but they are in fact the same thing.
To understand this counter-intuitive thought, let’s look at the number line:
Addition moves to the right on the number line, as in these examples:
Subtraction moves to the left, as in these examples:
Consider thinking of it like this: subtraction is addition of negative numbers. We just usually leave off the addition sign—but we don’t have to! These are all perfectly valid:
With a little practice, this makes dealing with positive and negative numbers much easier.
Adding positive numbers is moving to the right.
Adding negative numbers (subtraction) is moving to the left.
I will get into subtracting a negative from a negative in two posts. Next up: multiplication and division. After that: sign rules. Yes, you will finally understand why negative times negative is positive!
Open Your Calculator App
This isn’t feeling very intuitive for some of you, so open your calculator app on your phone and prove it to yourself.
Do 100 - 70 and get 30.
Now do 100 + (-70) and get 30.
What to Focus On with Little Kids
For young children, tactile objects that they can count are the way to start. Save the number line for when they’re old enough to handle a bit more abstraction.
The first light bulb moment for little kids is the trick of completing a ten. 9 + 7 = 16, and the way to think of this is to take what you need from the 7 to complete the 10, and then the answer is easily obvious. 10 + 6 = 16.
Once they have that, guide them, with questions if possible, to the realization that everything starts over with tens. 9 + 7 = 16, and 19 + 7 = 26, and 29 + 7 = 36, and so on. It’s beautifully predictable, endlessly repeating cycles.
When these ideas are solid, working with subtraction is an equally important, and simple, matter for drilling. If 9 + 7 = 16, what’s 16 - 9? Your kid may do the reverse in their head first, asking themselves 9 plus what equals 16? Or they may do 16 - 10 is 6, plus 1 is 7. Both are excellent ways to think about these problems and should be heavily praised.
When Your Kid Is Ready
Drilling is of crucial importance. These things should be second nature.
If you want your kid to be good at math, then by the time they’re ready for algebra, all of these basic arithmetic skills should be automatic and rote. The California frameworks and other educational strategies that don’t use memorization are shortchanging kids tremendously.
Why?
The reason why kids struggle with algebra is that their arithmetic skills are weak. If every single step of an arithmetic problem requires intense concentration and focus and cognitive energy, they will tire easily. They will have the opportunity to make a mistake at every stage.
But if your kids knows that 60 - 4 is 56 because they made the connection that 6 + 4 is 10 and 10 - 4 is 6 and that these cycles repeat over and over, then when they see 7x + 4 = 60, they’ll see 7x = 56 in that without intense focus and thought. And because you’ll have made sure they memorize the multiplication tables (justification will be in the next issue), they’ll know that x = 8.
The difference between a kid whose arithmetic is solid and one whose arithmetic is weak is one who can look at 7x + 4 = 60 and get x = 8 almost without thinking vs one who has to do multiple arithmetic steps, using a lot of energy and potentially making a mistake, at every point.
Part 2: multiplication and division, why they’re the same thing, and how understanding that lets you subtract negative numbers from negative numbers.







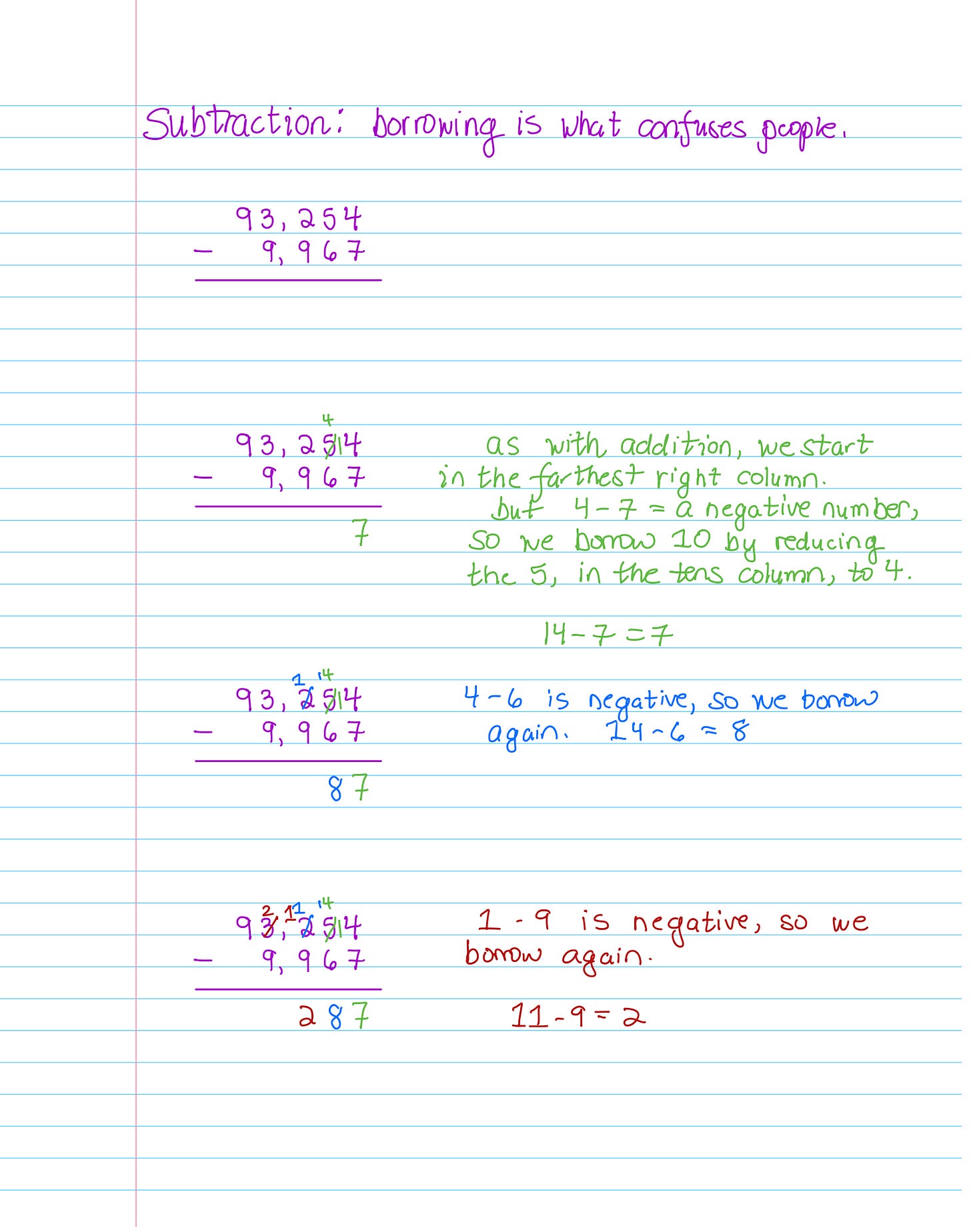
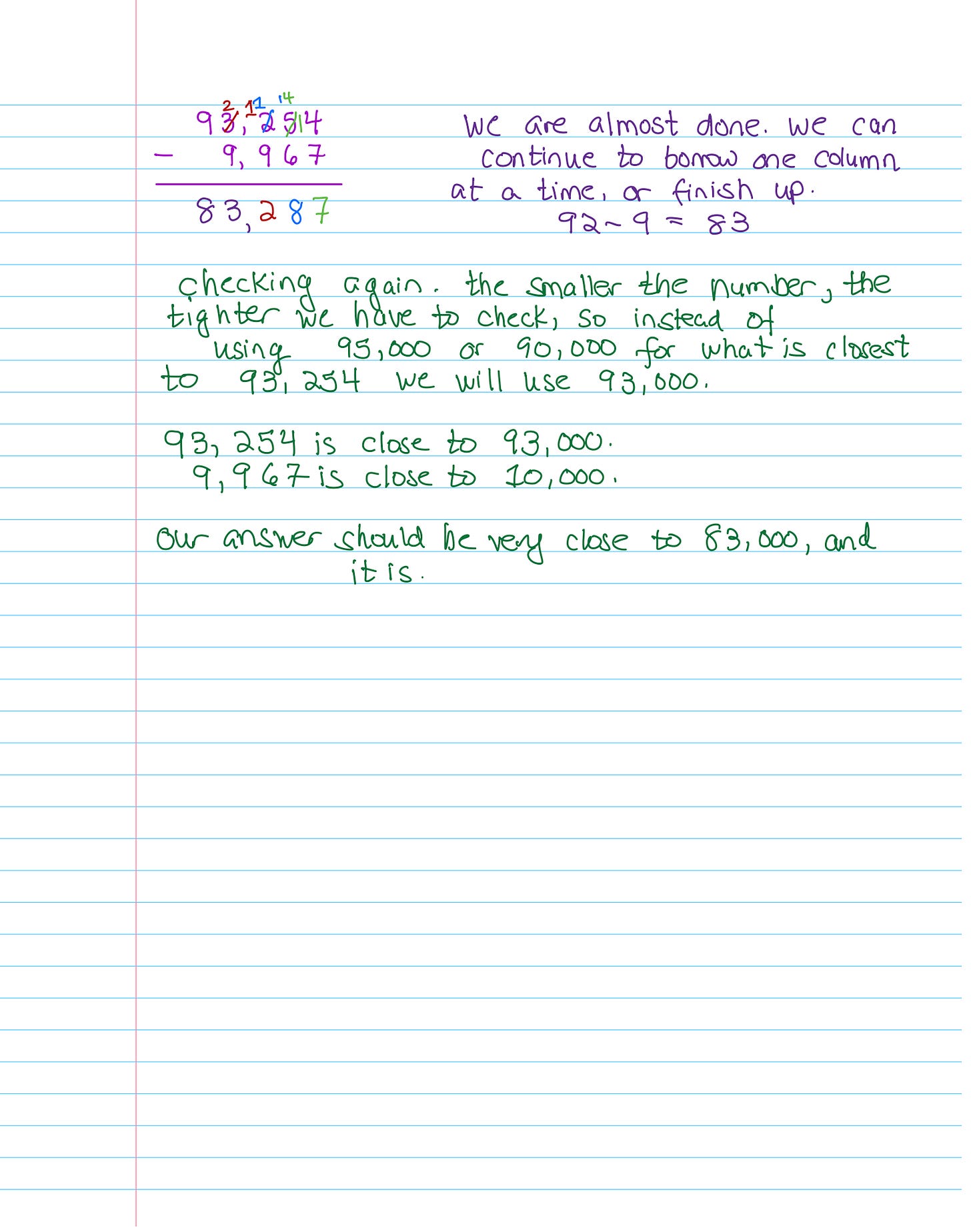









> But if your kids knows that 60 - 4 is 56 because they made the connection that 6 + 4 is 10 and 10 - 4 is 6 and that these cycles repeat over and over,
Notice that this is actually algebraic reasoning, even if it's not a formal algebra problem. I wonder if that also helps kids learn explicit algebra (on top of the effect that you are talking about here).
Haven't read through all this yet, but wondering how many tines you've swwn or heard "New Math" by Tom Lehrer.