Decapitating Meritocracy's Corpse

my review of Jo Boaler's book, "MATH-ish"

---

This post has a lot of pictures and thus is too long for most email clients, especially gmail. Click on the title above to read it on the Substack website.

---

Oh, Lord.

Time to review Jo Boaler’s book.

The book by the woman who dismantled the math curriculum standards of California — the largest market for textbooks in the United States — and in so doing fucked up math education for every American.

Yes, I read it. Every single word. Most of it on my exercise bike, where the rage could at least serve a useful purpose. I got the Kindle version so I could take screenshots for this review, and even managed not to throw my iPad across the room when I really, really wanted to.

I should probably not put this one behind the paywall, as everyone needs to understand what’s happening to our educational system, but I suffered in reading this, so I’m leaving much of it behind the paywall for the satisfaction of knowing that people who’ve paid for the privilege are the ones will benefit from my suffering.

Yes, I said suffering. No, I’m not being dramatic. You try reading about what you love most in the world being disrespected, demeaned, shat upon, and belittled and tell me that the word “suffering” is too much.

My snark muscles are fully loaded, and powered by rage.

Buckle up, y’all.

(Unless otherwise noted, all pictures are screenshots from the Kindle version of the book.)

This is one of the most frustrating books I’ve ever read, because she’s not completely wrong in every aspect of her arguments.

A lot of her recommendations would be helpful in individual classrooms for some kids and some teachers in some situations.

A lot of the ideas she advocates for are ideas that I use in tutoring, in fact.

They’re not all bad ideas.

The problem is that instead of a book about how elementary, middle school, and early high school teachers can do a better job of teaching mathematics—imparting number sense, making math something that kids enjoy and aren’t terrified of, helping more kids avoid math trauma and the sense that they’re “just not a math person”—she wrote a book about how to use mathematics classrooms to “address inequity,” re-engineer human nature, and turn kids into good little collectivists.

She also undercuts her own arguments—even some of the arguments she uses to support good ideas—in a number of places, often in ways that rise to the level of “massive self-own.”

This is going to be really long, because to attack her arguments I have to go into some depth about why and how I see things differently.

And that means telling you about two things for context: growth mindset, and the math wars.

Growth Mindset

Carol Dweck’s book, Mindset, posits a dichotomy between a “fixed” mindset and a “growth” mindset. Growth mindsets see struggle, mistakes, and even failure as an opportunity to learn and grow. People, particularly children, with growth mindsets believe that if they are flexible and persistent — keep trying, and trying in different ways — they can eventually learn anything. Fixed mindsets are characteristic of those who believe that people are born with a certain level of ability that is pretty much set in stone.

For one relevant (to me) example: people who believe that they’re “just not a math person” and develop a kind of learned helplessness — the kind of people who respond to my posts and Notes with things like “LOL it’s all Greek to me, you might as well be a space alien ha ha,” and proudly refuse to ever try — have a fixed mindset.

People who read my math posts slowly, more than once, and ask questions if they need to, but trust that they can in fact get it if they try — have growth mindsets.

(Obviously, most of us have fixed mindsets in some areas of our lives; these are not categories of simple, uncomplicated purity.)

Neuroplasticity, the fact that our brains are always making new connections, especially when we’re young, suggests that the growth mindset is the more accurate framing of human potential.

Dweck’s book discusses these two mindsets in engaging and interesting ways. I love the book and have found it to be extraordinarily helpful to me.

I will write about this at length someday, but I used to have a very fixed mindset. Fixed mindsets often result from trauma, and I had one to be sure. I read the book not long after it came out, and it gave me hope that I could heal from a lot of my childhood damage, make up for what I lost when I was being “educated” in a church basement, and otherwise that I had much, much, much, much more control over my outcomes than I had previously imagined possible. That book made it possible for me to believe that an internal locus of control was something I could learn to develop. I haven’t fully succeeded at this, by any means, but I’m absolutely unrecognizable to myself, in many ways, from before I read that book.

So growth mindset is, absolutely, a powerful and important thing to try to instill in children.

But, as with most powerfully good ideas, people started trying to figure out how to make money from it.

A lot of people developed a variety of ways to try to “teach” this in schools, and many of those ways failed. Kids quickly learned the right answers to give — it’s school, of course they want you to say on the quiz that you can learn anything if you try — and the various studies showed extremely mixed results.

Note that none of this means that growth mindset isn’t a thing, or that it isn’t a powerfully empowering idea that can help individuals develop an internal locus of control. It is, and it can.

I have a degree in mathematics hanging on my wall to prove it, in my case.

But not everything is suitable for teachers to teach at scale, in a classroom.

Some things can be learned from teachers. Some things can’t. Other things can only be learned by an older child or adult, on his/her own.

The kind of self-insight it takes to recognize a fixed mindset, want to change it, and then change it is probably not something that classroom teachers are likely to inculcate outside of a few individual one-on-one relationships.

And that’s ok.

She mentions growth mindset approximately every fourth sentence, which is a problem — again, not because growth mindset isn’t real, important, vital, and empowering. It is all of those things. It’s just not something that teachers can or should be wholly or mostly responsible for. It’s something that’s largely up to parents and kids themselves. Teachers can encourage the nascent seeds to grow, but I remain unconvinced that teachers can plant it, at least in most cases.

The Math Wars

This is going to be a ridiculously short and inadequate summary, just barely enough for my purposes in reviewing her book.

The traditionalist, classic math lecture is called “I do, we do, you do.” The teacher does a problem on the board, slowly and carefully, narrating each step. Then the teacher puts another problem on the board, seeking student input at each step. Finally, the students are set a problem to do on their own.

Rote memorization and lots of drilling is part of the traditionalist approach to math teaching. It also includes ability grouping — where some kids are put on a calculus track and others aren’t, or some kids are placed into remedial math and others into advanced.

The progressive side (the “reform math” people) believes in changing everything. Students should spend a lot of time tying mathematical learning to real life, and shouldn’t be presented with new mathematical tools or ideas until they “need them”. They believe in things like having students illustrate their thought processes, endless group work and discussion taking priority over everything else, and never grouping by ability. They often carry this to ridiculous collectivist extremes. The “I” in this screenshot is Boaler, referring to her university classes:

The school she cites most positively in this book, referred to as Railside, takes communism in the classroom to a ridiculous extreme:

I cannot imagine anything worse for the kind of smart, often introverted kids — especially boys, who are frequently years behind their female peers in social skills — who excel in traditional mathematical settings than making them responsible for everyone else’s learning, lest their own grades suffer.

Boaler is the heroine of the progressive side of the math wars.

My Take on the Math Wars

My take is that the progressive side is mostly wrong, but some of their ideas are good. There’s no need to reject a good idea just because some lunatic wants to apply it too broadly. Thus, some of their good ideas can and should be incorporated into a traditionalist approach.

There absolutely are a LOT of kids who could go on to study math at a high level, and have math-based careers open to them, who wash out of math in the current set-up. Needlessly. It absolutely happens, and it wastes talent and the potential for innovation.

Adolescence is the rockiest time of life, and if every kid who hits a rough patch between sixth grade and high school graduation finds the door to a mathematical career slammed shut — which is sometimes the case, especially for poor kids — that’s a problem we should be solving. (More on this later.)

One example of a “reform math” idea that’s worth incorporating is valuing struggle. Math is hard. Often it’s the very first subject that a smart kid, especially a smart kid from a two-parent home that values education, has to struggle in. Kids who have never developed persistence or resilience in academics — because they’ve never struggled with anything academic before — often decide that they’re “not a math person,” and this is tragic. Math is the most beautiful, intriguing, amazing part of life as far as I’m concerned, but I only know that because I had persistence externally forced on me. (I had to succeed or I’d have a mountain of debt and no way to pay it off; failure was not an option.)

Substacker

Jason Jonker

wrote one of the most insightful, honest essays I’ve ever seen. When he had to struggle with something academic for the first time, he took the story that many smart kids — kids who are smart enough to fully convince themselves of bullshit — tell themselves and used it to craft a narrative of why his being “bad at math” was actually evidence of his great personal virtue. (He learned math later, when he stopped feeding himself that line of poppycock.) I will forever be grateful to him for writing that post.

So yes, teaching kids that struggle is part of the process? Good idea. Important. Helpful.

Having them conceptualize struggle as a pit in which they should do interpretive dance? Not so much.

No, I’m not kidding. (Would that I were.)

Valuing struggle is important, to help students stop being afraid of it.

Making a goddamn dance party out of it is not.

Another important idea that the reformers get (mostly) right is presenting concepts with multiple approaches, which she calls “mathematical diversity”. For example, some kids will grasp that multiplication is “faster adding,” while others will have their light bulb moment with something more visual, like an area model:

When I was an undergraduate, I would go to class. Then I would often come home and watch two or three YouTube videos of professors lecturing on the same topic, downloading their powerpoint decks when available. The overlap of approaches — the same thing explained differently, often very differently — was my best tool. And it worked very well.

The most frequent thing I hear in math tutoring sessions is some version of:

“Oh. Why didn’t my teacher just say it that way? That makes sense.” This occurs when I’ve simply explained whatever it is they’re not getting, in different words than the words their teacher used.

So yes, multiple conceptual presentations is a good idea, at least in some classrooms.

The reformers despise ability grouping (the concept of remedial, regular, and advanced math classes) and on this they have one, and only one, small point. It is true that in some districts, to get on the calculus track you have to take Algebra 1 in eighth grade. And to do that, you have to take pre-algebra in seventh grade. And to do that, you have to do well on a test that you’re given in sixth grade. And ridiculously, these tracks sometimes have no additional on-ramps.

Sixth grade is the year when many kids hit puberty. So a girl who takes a test the week she gets her first period or a boy who takes the test when distracted or distressed because he’s noticing girls, or other boys, in a new way and doesn’t do herself or himself justice on a single test could, in some districts, be derailed from a math track.

That’s ridiculous. Nothing an eleven-year-old does should be that high-stakes.

Kids with involved parents will often find their way back onto the math track. Tutoring, summer school, changing schools, a summer camp, or even something as simple as having a parent request re-testing — these are all things that kids from poor or single-parent families may not have available to them.

But all this means is that the concept of “ability tracking” should be more flexible in the districts where it’s presently quite rigid. Some kids get interested in math later. Some kids have a rocky time in elementary or middle school but settle into themselves as they get older.

Some kids have a beloved grandparent die, or must deal with parental divorce, and have a bad year. Rather than being locked into a track from a very early age, multiple opportunities should be available for kids who want to take more challenging math in high school — or even potentially might want to — to get the needed prerequisites under their belts.

That’s their one legitimate point, and it doesn’t even apply in all districts. I’ve heard of kids being allowed to use a summer math camp in place of pre-algebra, to take Geometry and Algebra 2 simultaneously in tenth grade, or other such flexible approaches.

Beyond that one point, their arguments against ability grouping are horseshit.

Devil’s Advocate Hat Off

I’ve tried very hard to be fair here about what the “reformers” get right.

But instead of “reform math” types trying to improve the things that need, or at least could benefit from, some improving — they are trying to change everything for everyone.

And their efforts are driven by political bullshit, so of course they’re succeeding.

Here are Boaler’s theses as I understand them, and why they’re somewhere from partially, to mostly, to entirely, batshit.

Thesis 1: Mathematical Diversity (Mostly Not Batshit)

One of her primary theses is that students benefit from “mathematical diversity”. In some parts of the book, she uses the word diversity to simply mean presenting students with multiple ways of looking at concepts.

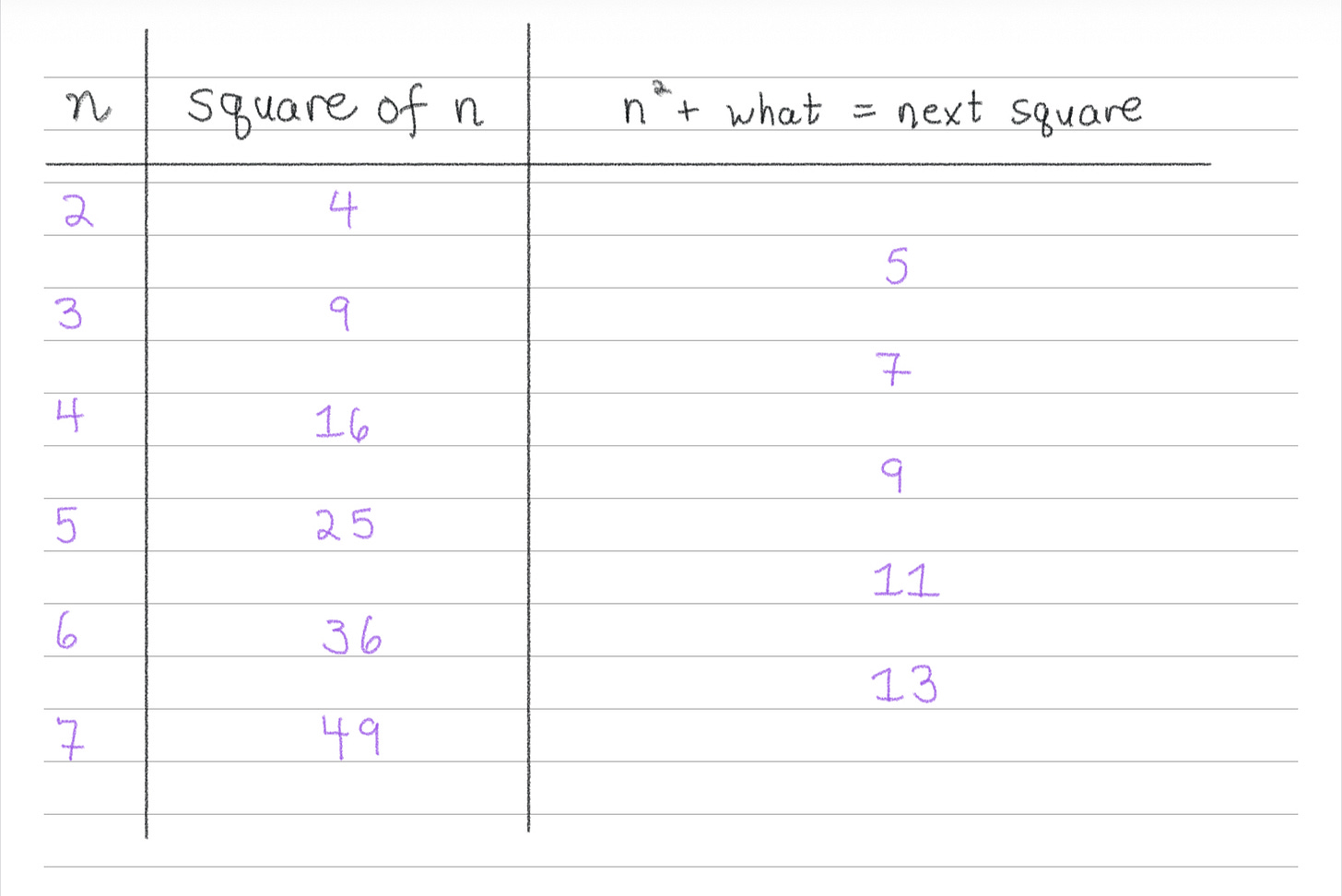
For example, instead of teaching the merely abstract — that squaring a number is multiplying it by itself — using visual representations to show that squared numbers can be represented as squares:

In the next sentence, she commits a massive self-own due to lack of clarity:

“…square numbers come from the addition of odd numbers,” just above an illustration that includes 4 + 5 = 9, is confusing. Four is not an odd number.

I think what she meant to refer to was a pattern among the squares of the natural (or counting) numbers, the 1, 2, 3, 4, 5….and so on. That pattern looks like this:

(Yes, it does go on forever.)

Both the visual representation of squares and the corresponding pattern are valuable, helpful, and very cool things to point out—and surely things that help some people make mathematical connections and see proverbial light bulbs.

But this pattern is not one that I should’ve needed to grab my iPad to verify was the pattern she was referring to.

In other parts of the book, she uses the word “diversity” in the political sense — to try to force “equity,” reduce “status,” and to blame disparities in achievement on systemic injustice at all times.

Conflating the two makes the book an absolutely maddening reading experience, and throws into sharp relief one of its many contradictions. To keep them separate here, I will make “Mathematics is a Tool To Force Equity” a separate thesis.

But mathematical diversity in the sense of multiple perspectives and approaches being offered, so that students have more opportunities to gain understanding?

That’s not a bad thing, and some of the ideas for doing so—especially capitalizing on the fact that little kids are often very visual and tactile, by offering visual approaches and manipulable items in addition to the purely abstract ideas of numbers—are good.

Thesis 2: Ish Should Always Be Valued (Mostly Batshit)

One of her theses is that answers in the ballpark — what we call estimation and she calls “ish” — is highly undervalued, and should be much more highly valued, and often accepted as a correct answer. She supports this with the idea that in real life we usually don’t need a high level of precision.

Well, it depends.

Take this page of examples from the book:

Each and every one of these demands precision in some circumstances. Having the other party sign a contract the day before their 18th birthday matters quite a lot insofar as enforcing that contract. “Half” of a cookie had better have a very precise meaning if you’re dosing the insulin for a diabetic toddler. How warm is it outside? Being ten degrees off can mean death for a pet left unattended too long.

How many of us want our paychecks, medication, utility bills, or other things that matter calculated by someone using “Math-ish”?

I could go on, but you get the point—the need for precision is context-dependent.

She also commits a Texas-sized self-own in one of her applications of “ish”.

The second screenshot shows a glaring error. Multiplying the numerator and denominator by the same number does indeed keep the same fraction, but she fails to explain why, and she also doesn’t do it here. 3/4 x 2 = 6/4, not 6/8. Likewise, 3/4 x 3 = 9/4, not 9/12. What she meant to show was a process that’s always permissible: to multiply the numerator and denominator by the same number, not just the numerator as shown here. She meant to multiply these fractions by 2/2 and 3/3, not 2 and 3. What she failed to explain (talk about a self-own…) is why this is always permissible, and often helpful.

A fraction of any number over itself — 3/3, 2/2, 179/179, etc. — has the value of 1, and thus multiplying any fraction by it is the same thing as multiplying that fraction by one. 3/3 is three thirds — a pie you split into thirds, but otherwise left untouched. Nobody ate or took away a third; it’s just sitting there, one whole pie, cut into thirds. (Likewise for all other cases of the same number in both numerator and denominator of a fraction.)

This is always permissible because multiplying by 1 doesn’t change the value. It is often helpful because it can create a like denominator, making the numerators easier to deal with.

But when I first saw it, it was in the form of a Twitter screenshot that was going around to mock her, and it took me a couple of minutes to catch the error. Why? Because I knew what she was trying to do, so I mentally filled in the blanks. I did that because I am mathematically fluent and have strong number sense.

Kids don’t, and this kind of thing could be profoundly confusing.

There are some places where “ish” is ok and even helpful, sure. But it tends to promote sloppiness that must be guarded against.

In another place in this section, she creates another massive self-own.

Gosh. I wonder if she instantly knows that 8 x 30 = 240 because of many, many repetitions of the rote memory fact that 8 x 3 = 24 and that multiplying by a factor of 10 (as 3 x 10 = 30) puts a zero on the end, turning 24 to 240?

Nah, probably that one stuck with her because of interpretive dance.

Thesis 3: Metacognition Is Crucial For All Mathematics Learners (Mostly Batshit)

Metacognition is essentially thinking about your thinking.

A few examples of my own metacognition: I know that when I am working on a number theory problem, there’s a point where I start to see the form of the problem. I see whether it should be a proof by induction, a proof by contradition, or some other form. Or if I start off having a good sense of the form, then it’s when my errors start to feel closer to something solid. When I get there — when I am no longer just screwing around, but can actually start to sense what the solution will look like — I recognize that the obsessive part of my brain will take over. That is the point where I had better put my iPad away if I can’t afford to do nothing else for the next several hours.

Additionally, I know that my best strategy for learning something totally new in mathematics is to read a summary of the method, then to watch a high-level YouTube lecture video, then to write some kind of pseudocode (pretend that I’m going to code something to get a computer to solve it and write out roughly the form the code would take), then read a math book in depth, then to watch a more in-depth YouTube video, then to refine my pseudocode. Usually by this point I am ready to do whatever I need to do with it. (A third example of metacognition on my part follows in the section on thesis 4.)

Metacognition is a very helpful skill for learning math, but only in older kids. In little kids, asking them to think about their thinking is too much. They barely have a concept of themselves as separate beings from their parents, and she wants them taught to think about their thinking—to learn how they learn. In bigger kids (from about 8 to adolescence) some kids are ready for this, and some aren’t. Boys tend to mature more slowly than girls, and metacognition is something that males often take longer to grasp. So this is another case of “maybe helpful, maybe not.”

One of the metacognitive techniques she proposes is having students reflect on their own learning in journals. This is a feminized practice, and I do recognize the possibility of some benefit. Asking tutoring students what they think they understand best, and why, is something I always do, to good effect. Though verbal instead of written, it’s kind of the same thing—but as part of standard classroom instruction, especially mandatory and graded, it strikes me as ridiculous. An optional activity, available as one of many extra credit choices? Sure. Mandatory? Fuck no.

Imagine a boy who loves and is very quick at math having to stop and think about what to write in his metacognition math journal. It’s not hard to imagine the teacher comments on an entry like “I think I’m doing great at math because I get the right answers and it’s fun,” especially coming from a white boy (she’s obsessed with racial and gender “inequities”).

Thesis 4: Conceptual Learning Trumps Procedural Learning (Partially Batshit)

One of her (good) ideas is that students grasp mathematical concepts at a deeper level when they are guided, encouraged, and helped to ask “why” questions — why does it work this way? What tools work, and why do they work?

And yet she never, ever, ever asks this question herself about any of the “systemic inequities” she seeks to remedy by socially engineering kids through math classes. (More on this later.)

She is also firmly committed to the idea that understanding the concepts behind why something works should precede learning the procedures and algorithms, every single time.

That’s a blanket statement with which I disagree strongly.

Your kids should memorize their multiplication tables, even if their schools do not require this. They should know them cold, backwards and forwards.

For some kids, a deep conceptual understanding in advance of this will be helpful, motivating, and empowering.

For other kids, it will work the other way. For some kids, the most helpful thing you could do would be to reward them for being able to recite their times tables perfectly. Then, and only then, the fluency of having so many answers memorized and at the ready will make them feel like superheroes, which will provide the needed confidence and enthusiasm to help them begin to grasp why multiplication works.

When I was majoring in mathematics, it worked the latter way for me. I needed to see the proof for the mathematical tool I was working with — the chain rule in calculus, Rolle’s theorem, the squeeze theorem, or whatever it was — but I needed to see it only after I’d gotten a few right answers. Before then, it just confused me and made me anxious. Applying the algorithm by rote and on faith first was crucial for me. Knowing that I was already using a tool, and using it correctly, made it possible for me to understand the concepts behind it.

The various tutors and professors I spoke to as an undergraduate told me that my need in this area, while unusual, was not unheard of. They’d all had students like me before. My suspicion is that those students were either anxiety disordered in general, like me, or perhaps had a high level of math anxiety (which is something that applies, I would venture to guess, to more people than it does not). Removing the fear of failure by allowing a student to get a few right answers first can make conceptual understanding much more likely to follow.

More on Thesis 4

She argues, correctly, that one thing that characterizes good “number sense” is the awareness that you can always split a number into others numbers. Professors of education call this “groupitizing” or “subitizing”.

She is right about this; that understanding is crucial. The mental flexibility to split a problem into smaller or more easily handled parts is a vital skill for number sense.

In my series, “How to Not Suck At Math,” I argue in part 3 that everyone should learn the Fundamental Theorem of Arithmetic at as early an age as possible. (The first five parts are not paywalled and can be found here.) For most kids, it will be possible around the time they’re learning their multiplication tables.

That theorem explains that every number fits one of two categories: it either is prime, or it can be decomposed (split) into a unique (up to the order) combination of prime factors.

For example, 11 is prime. 11 can only be evenly divided by 1 and itself.

10 is not prime. Ten can be split into the prime factors of 2 and 5. Multiplying 2 and 5 (or 5 and 2; the order isn’t considered to violate the uniqueness) will produce 10. It will never produce any other integer (whole number), and 10 can never be broken down any further into integer factors.

Learning to think in terms of prime decompositions has been a game changer for me and many kids I’ve tutored.

I show in part 4 of my series how this approach makes everything simpler, easier, and more flexible when it comes to problem-solving.

So yes, groupitizing—in some form, ideally several forms—is a crucial mathematical tool, but how she goes about this discussion reveals a perspective that is jaw-droppingly stupid. She is utterly obsessed with “taking down a peg or two” anyone who excels at math in traditionalist settings, to the point of making herself look ridiculous.

“Students were high-achieving not because they knew more, but because they approached numbers differently.” As if the ability to approach differently doesn’t stem from knowledge!

In other news, Gordon Ramsey was high-achieving not because he knew more, but because he approached cooking differently.

Stephen Curry was high-achieving not because he knew more, but because he approached shooting the basketball differently.

Groupitizing (or some form of flexibility in understanding how to break numbers apart and put them back together correctly) is crucial.

But does the entire bloody mathematics curriculum need to be up-ended so that students can work in groups, reminding each other about grouping?

Or does this need to be an idea that teachers introduce earlier, reinforce more often, and remind students of regularly as they teach traditional mathematics?

Thesis 5: Mathematics Is A Tool To Force Equity (Totally Batshit)

This was the most extraordinarily frustrating part of the book. She sees mathematical education as a tool for equity, and this is based on the shallow, stupid assumption that every disparity between groups is based on “systemic inequity.”

The political agenda here is obvious and disgusting. In one section, where she talks about the racial makeup of students on the calculus track — kids who get to take calculus in high school — she mentions that 46% of Asian students, 9% of Latino, and 6% of Black students take calculus in high school. She leaves out White kids entirely. Why?

I looked it up — 18% of White kids take calculus in high school. About 40% of the rate of Asian kids. Kinda pokes a hole in that “white supremacist” narrative, huh?

By the way, the percentage of kids from two-parent homes in the US is 81% Asian, 70% White, 55% Latino, and 33% Black. The kids from two-parent homes, in every group, parallel that group’s mathematical achievement.

It’s almost as if stability at home has something to do with educational success.

Her obsession with equity is revealed in the many classroom changes she proposes that are, in a word, feminizing. This is another situation that’s maddening, because they don’t have to be. Some of them—like the metacognition example mentioned—can be good for boys and girls alike, but it’s crucial to make these things optional. More choices, not fewer.

But no, we can’t do anything sensible like just improve known procedures. Instead, we need to focus on group dynamics, collectivism, and destroying any attention to individual merit or rewarding individual effort.

A school she cites as ideal spends the first ten weeks of the year focused on “respectful interactions”.

She also objects to competition. Which, again, is a case where more choices, not fewer, are needed. Everyone doesn’t learn well in a competitive atmosphere. A balanced classroom will offer opportunities for both competition and collaboration.

Rather than expanding thinking in a way that includes everyone, it’s all about figuring out what helps boys, and girls of more masculine-typical temperaments, succeed, and taking it away. Why? To shape future politics, of course.

The obsession with group dynamics is insane, and taken to the most ridiculous extremes. As already mentioned, giving all group members one grade to make them all responsible for each other’s learning is a recommended practice.

How in the hell are teachers, parents, or others supposed to know how kids are doing when they’re graded by group membership?

Why not just grade them by skin color and chromosomes, for fuck’s sake?

Here’s a rubric of proposed group roles. Note the intensity of the social and emotional aspects, and tell me this isn’t designed to cut advanced students off at the knees. Instead of getting an A in math for knowing math, good students must have solid social skills (which strongly favors girls) to succeed at math. Absurd.

Thesis 6: Less Rigorous Forms of Math Matter Just As Much (Total Fucking Insanity, 100% Grade-A Batshit)

She proposes what she calls “data science” as an alternative to algebra, trigonometry, and calculus.

This is, first of all, not data science.

Source: me. I’m a data scientist at a Fortune 500. I use algebra every day, and use the principles from calculus all the time. I’ve even used trig once or twice.

In this section, she again self-owns:

I know someone whose half-brother left half a leg in Iraq, in the service of our country.

He is literally a half-sibling with half a leg.

Data literacy is what she’s talking about — not data science. Data literacy is understanding what a survey is, what a population is, and having some sense of what methods are used. Knowing enough to ask questions like “Who funded this study?” and “What was the sample size?”

Data science involves being able to take data and come to reasonable, math-based conclusions about them. Data science requires understanding math through calculus, as well as statistics, logic, and the kind of clear thinking that one learns from coding.

Conceptualizing data science as an alternative to algebra, trigonometry, and calculus is like conceptualizing building houses as an alternative to carpentry, plumbing, and electrician work.

Thesis 7: Only Bigots Disagree (Cynical, Dishonest, Total Batshit)

Boaler’s work is controversial as part of the math wars.

But it is controversial for other reasons, too. She has been accused of faking data, including much of her data asserting that “reform math” works. Stanford investigated and declared these charges baseless.

I don’t know what to think about that. It is hard for me to believe that Stanford would throw out data that promotes a Woke view of math, even if it was in fact fabricated.

But on the other hand, it’s not hard for me to conceptualize how to set up a study that would show these methods working very well in an individual school. So it’s entirely possible to me that all of the claims she makes about her studies are true in that sense.

These methods are new, interesting, and different. If I wanted to set up a study to prove that these methods were superior, I would first find a school that has a demoralized math department, filled with kids who are disadvantaged — not necessarily disruptive, troubled, or delinquent, but just disadvantaged.

Kids who are basically good kids, but come from homes with little or no books. Kids whose single parents can’t spend much time helping them with their homework. Kids who were discouraged by their past failures but wanted to do better because they weren’t jaded yet.

Then I would give them special attention by including them in this new, exciting type of math pedagogy, and I would either teach it myself or carefully choose an effective, passionate teacher to put in 100% effort.

It would probably work, at least for the length of a study.

So is her data faked? Or was her study carefully planned to show success where she wanted it to? I have no idea, and I don’t really care.

What I do care about is this — it is entirely possible to disagree passionately with her and not be racist, sexist, or anything else considered morally problematic.

But not in her mind.

Notice that she just assumes that her methods promote “true understanding,” which is quite a claim to make so flippantly.

In her mind, the only way that anyone can disagree with her is by a desire to maintain their “special” status as superior at mathematics.

Speaking as someone with a math degree and a job doing math at a Fortune 500 — not only do I not see math that way, I go to great lengths to communicate my profound belief that most people can go much, much farther with mathematics than they think.

But I disagree with her, so I’m merely threatened by “equity”.

So, likely, are you.

What a magnificent, grandiose, gloriously stupid pile of horseshit.

In Conclusion

There are some good nuggets here — some ideas that are potentially valuable, some of which I already use in tutoring, and some of which I would in fact recommend to homeschooling parents.

But none of them — absolutely none of them — warrant massive changes to all classrooms in all situations, and certainly not re-working the mathematical curriculum standards for the entire country.

The eleventy-ninth (how’s that for “ish”) frustrating thing in this book?

She freely admits that what’s good here can be used by individual teachers in individual situations!

Example: nobody has to change mathematics textbooks to give the following assignment as an additional approach when studying multiplication and the concept of area.

She freely and openly admits that “countless” teachers use her ideas to great effect all the time, without changing standards!

This whole thing is the usual Woke formula.

1. Take a real problem.

2. Instead of addressing it in a reasonable way that works to help the people who need help without destroying what works well, destroy everything, especially that which does work.

3. When this doesn’t work to make everyone exactly equal, Communist even harder.

It is a real problem that mathematical education is often conducted in a way that causes kids with real potential to wash out much too early. We do need more ways for kids who want to study math to a high level to be able to do so, without having to have a perfect, unblemished scholastic record as far back as sixth grade. Life is messy, and not every kid with the potential to take calculus is able to follow the great-score-on-sixth-grade-test —> pre-algebra in 7th grade —> Algebra I in eighth grade —> Get through Algebra II, Geometry, Trigonometry, Precalculus, and Calculus in high school track, for myriad reasons.

And yes, there are plenty of teachers who could do a better job—presenting multiple approaches, working harder at making math accessible for kids — and plenty of kids who face frustration and an unwarranted sense of failure when the usual explanation doesn’t click for them the very first time.

But the answer to all these things is not collectivism, making kids responsible for other kids’ learning, ending rigor in favor of “ish” and group dynamics, setting up a situation where math scores are a proxy for social skills, and cutting off all possibility for advanced kids to pursue their potential to the fullest.

Just as kids with learning and other disabilities are entitled to accommodations, so are unusually bright kids.

They matter, too.

If your kids are in public school, you should get them out if you can.

If you can’t — and I do know that everyone can’t — you should supplement their mathematics, at the very least, at home. You cannot count on the schools to do anything more than train your kids in collectivism now.

This is where we are.

I’m still frustrated AF that this Woke nonsense is setting the standards for the entire US selection of textbooks now (the textbook publishers all go by California standards, to appease their biggest customer) but at least I got to say why.

This is one of the longest things I’ve ever published. I can’t imagine more than a few people made it to the end. If you’re one of them — thank you.

Thank you very much.